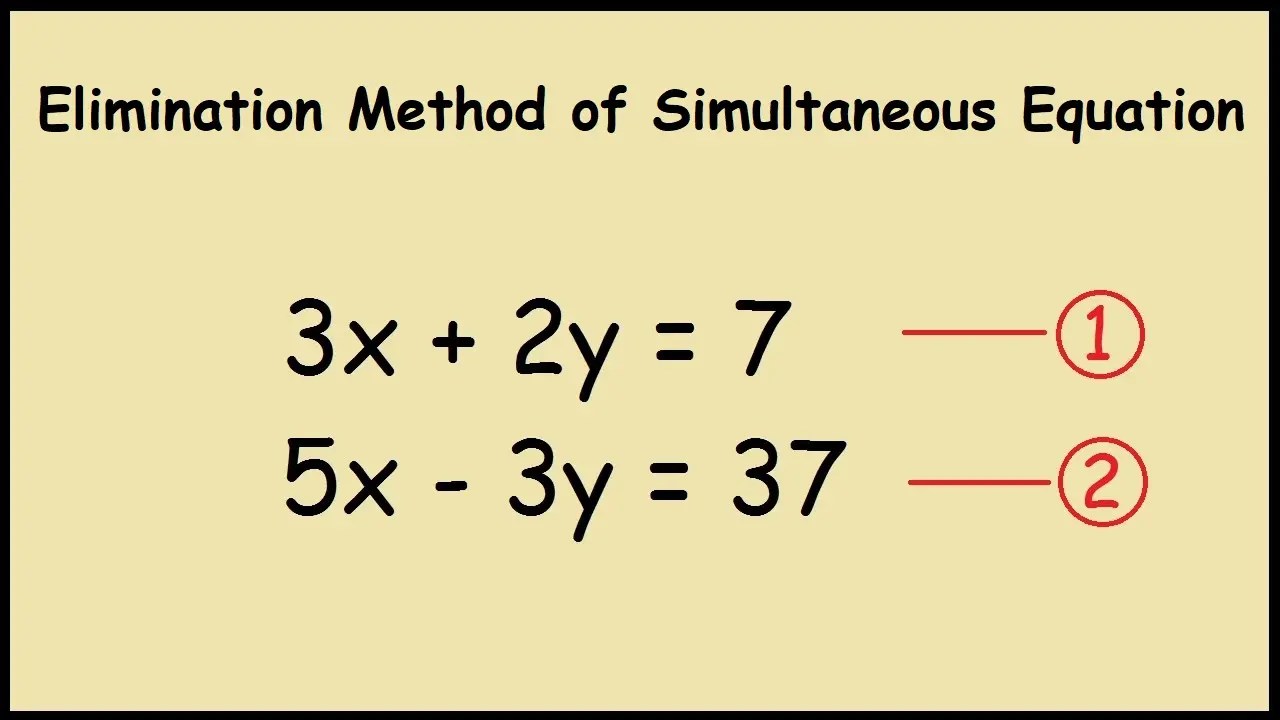
Simultaneous equation is quite an easy mathematics to solve if you are familiar with the required steps which has been broken down below. Note that there are two methods of solving any simultaneous equations either in WAEC, NECO, JAMB or any examination or test you are tried on this topic.
Simultaneous equation is one of the most common topics in mathematics that the West African Examination Council, WAEC test candidates for every year. You will either see it as an objective question or as a theoretical question and sometimes it could appear on both sections.
When simultaneous equation appears under theory section, most likely, you would be mandated to attempt the question using Graphical method because the body wants to test your in-depth understanding on this maths topic. Not to worry, we have broken down the steps on how to solve simultaneous equation graphically, it would assist candidates or students in a long way in achieving the full score awarded to the question.
For the sake of this post, i will be putting you through the simple and complete breakdown on how to solve simultaneous equations using Elimination method.
Ideally, there are 2 methods of solving simultaneous equations;
- Graphical method
- Algebraic methods (Substitution and Elimination)
I will be citing some examples on how to solve simultaneous equations using The Elimination Method.
Example 1:
2x + 3y = 12 —- (1)
5x + 2y = 8 —- (2)
Firstly we look for a way to eliminate either the x variable and coefficient (2,5) or the y variable and coefficient.) (3,2).
In order to eliminate either the x or y variable we make sure they have equal values. Now let’s eliminate the x variables to make y stand alone. The only way to make this possible is to take the coefficient of x which is 5 in equation (2) & multiply it with the whole of equation (1), and also take the coefficient of x which is 2 in equation (1) & multiply it the whole of equation (2).
5 2x + 3y = 12___(1)
X
2 5x + 2y = 8____(2)
We multiply equation (1), (2) by 5 and 2 respectively….
:. We have;
10x + 15y = 60____(3)
10x + 4y = 16____(4)
Now we can safely eliminate x variable and coefficient.
:. 10x-10x+15y-4y=60-16
:. 15y – 4y = 60 – 16
:. 11y = 44
We divide both sides by 11 to make y stand alone
:. (11y)/11 = 44/11
:. y = 4
Example 2;
4x – 2y = 10
x + y = 4
Multiply x + y = 4 by 2 to give 2x + 2y = 8
4x – 2y = 10
2x + 2y = 8
6x = 18
x = 3
You are to add the two equations which “eliminates” the 2y (since -2y + 2y = 0) and leave 6x = 18 which, after dividing both sides by 6 leaves x = 3
Therefor; 3 + y = 4
y = 1
Then you replace x in one of the equations with the value 3. (in this example, doing this in x + y = 4 is simpler than in 4x – 2y = 10)
4x – 2y = 10
(4 x 3) – (2 x 1) = 10
12 – 2 = 10
After getting your x and y variables, always confirm the correctness of your solution by checking the values for x and y in the other equation.
Did you find this simple to understand? if you have questions on how to best solve simultaneous equation with Elimination method in WAEC or any examination, please reach us via the comment section below and we shall respond accordingly.
Kindly share this post using the social media buttons on your screen so other students could also benefit from it.




